Introduction
In finance, the term discount rate is used in two contexts. One is, where the discount rate is taken as the rate used to discount the future cash flows to present values. The discount rate also refers to the interest rate at which the central bank extends short-term loans to commercial banks and other financial institutions. So, in this article, we will look at the discount rate as the rate used for discounting. We will understand the discount rate calculation and also look at how the discount rate is related to the net present value, time value of money and the internal rate of return.
What is the Discount Rate?
The discount rate is the rate of return which analysts use to discount the value of future cash flows to calculate the present value of an investment. Therefore, a discount rate on investment helps to analyse if the investment made is worth the time and money.
The investment’s profitability is determined by comparing its cost to the future revenue it generates. The discount rate is calculated when the future cash flow value is divided by the present value, with the reciprocal number of periods in power subtracted by one.
Formula
| Discount Rate | = (Future Value/ Present Value) ^ (1/ n) – 1 |
- Future Value: Show the value an investment will hold at a specific future date.
- Present Value: It represents how much the value of future cash flows holds at the present date.
- n: Refers to the time
Example:
In this example, we will calculate your portfolio’s discount rate, assuming it has grown from $20,000 to $35,000 in 5 years.
| Future Value | = $35,000 |
| Present Value | = $20,000 |
| n (number of periods) | = 5 Years |
Calculation of Discount Rate
| Discount Rate | = (Future Value/ Present Value) ^ (1/ n) – 1 |
| = (35,000/ 20,000) ^ (1/5) – 1 | |
| = (1.75) ^ (1/5) – 1 | |
| = 1.118 – 1 | |
| Discount Rate | = 0.118 |
The discount rate or rate of return on the portfolio is 11.8% or approximately 12% annually.
Different Perspectives of Discount Rate
Risk-Free Rate
The risk-free rate of return is the rate of return on the investment with no risk of financial loss. The discount rate considers the risk factor in the investments. A risk-free rate of return acts as the baseline for comparing numerous investment opportunities. The risk-free rate is a component in the calculation of the discount rate. If we calculate the discount rate using the CAPM model, we take the risk-free rate as a base and then add a risk premium multiplied by Beta to get a discount rate.
The formula of CAPM:
| Return on investment | = Risk-free rate of return + Beta * (Risk Premium) |
Hence, we can say that the discount rate is the minimum required rate of return for an investment considering both the time value of money and risk. The risk-free rate is a component of the discount rate used in financial analysis to determine the present value of future cash flows.
Hurdle Rate
In finance and accounting, the hurdle rate represents the minimum rate of return required on a project or investment. Investors and businesses use the hurdle rate in investing, business projects, purchase of assets, etc.
The cost of capital, risk tolerance, and opportunity cost of the company influence the hurdle rate.
Businesses evaluate investment opportunities by comparing the discount rate and hurdle rate. If the discount rate exceeds the hurdle rate, companies take on the investment. But if the hurdle rate is more than the discount rate investment, the investment opportunity is generally rejected or is subject to further analysis.
Weighted Average Cost of Capital
The weighted average cost of capital (WACC) is the average of the total cost of capital raised from debt and equity. WACC shows the minimum rate of return a company must generate from the investments.
In the discounted cash flow analysis, the weighted average cost of capital is used as the discounted rate because it reflects the overall cost of capital and the minimum return a company should earn to satisfy the investors.
| WACC | = [Cost of Debt * Debt Percentage * (1-Tax Rate)] + [Cost of Equity * Equity Percentage] + [Cost of Preference Stock * Preference Stock Percentage] |
Break-down of the WACC formula:
| Debt Percentage | = Total Debt Capital/ Total Capital of the Business |
| Equity Percentage | = Total Equity Capital/ Total Capital of the Business |
| Cost of Debt | = (Total Interest Expense/ Total Debt) * 100 |
Note: The formula shows the Pre-Tax Value of Cost of Debt. We multiply the Pre-Tax Cost of Debt with (1- Tax Rate) to calculate the Post-Tax Value of the Cost of Debt.
| Cost of Equity | = Risk-Free Rate + Beta * (Annual Market Return – Risk-Free Rate) |
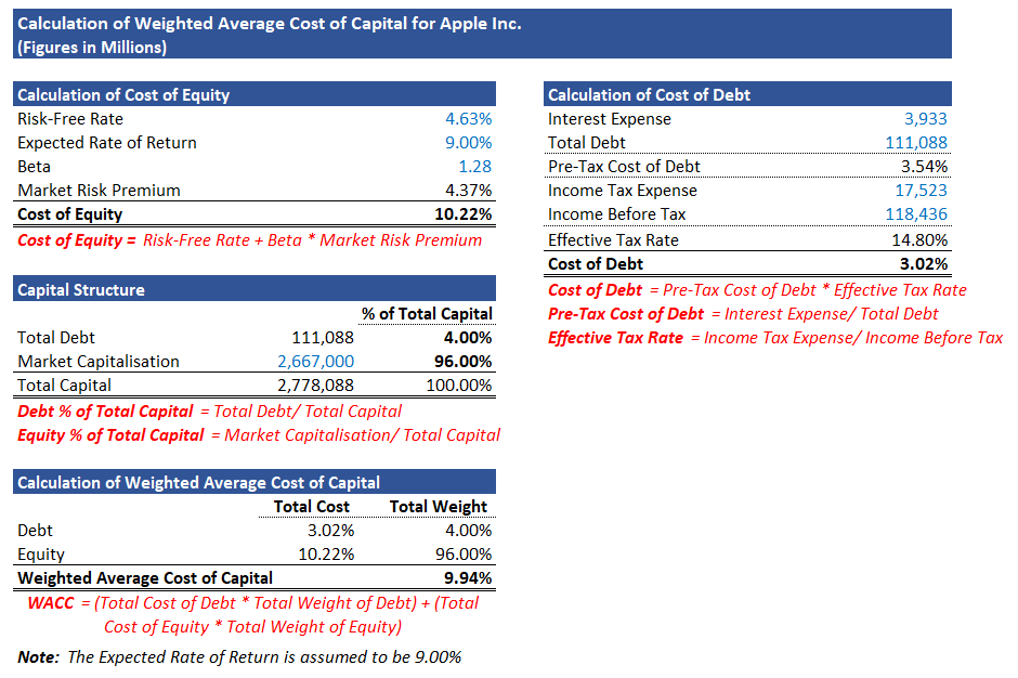
Calculation of Weighted Average Cost of Capital of Apple Inc. for 2023
The data for the WACC calculation is taken from the 10K Annual Report of Apple and Yahoo Finance.
- The Expected Rate of Return is taken as: 9.00%.
- For the calculation of the value of the Post-Tax Cost of Debt, the value of the Effective Tax Rate is considered which equals 14.80%.
- The Risk-Free Rate is taken from 10-year Treasury Rates as 4.63%.
Sources:
Financial Data:
- 10K: https://s2.q4cdn.com/470004039/files/doc_earnings/2023/q4/filing/_10-K-Q4-2023-As-Filed.pdf
- Yahoo Finance: https://finance.yahoo.com/quote/AAPL
Risk-Free Rate:
The Weighted Average Cost of Capital of Apple Inc. for 2023 is 9.94%.
Relationship Between Discount Rate and Net Present Value
Net Present Value (NPV) is the financial metric that measures the total investment opportunity with the help of cash inflows and outflows of that investment or project.
The discount rate is a vital component for the calculation of the net present value(NPV) of an investment. In the NPV calculation, the discount rate represents the rate of return on the investment. The discount rate is also used to bring the value of future cash flows in terms of present value for analysing the investment.
The higher discount rate can result in a lower value of NPV, as future cash flows are heavily discounted. While a lower discount rate produces a higher net present value for the investment or project, making it more attractive to investors.
Therefore, the discount rate plays a crucial role in determining the profitability and feasibility of an investment project through NPV calculation.
How is the Discount Rate related to the Time Value of Money?
The fundamentals of finance belong to a few core topics, and the time value of money is one of them. The time value of money talks about the worth or value of money considering the time horizon. It states that a dollar earned today is valued more than a dollar earned tomorrow. The value of money diminishes over time because the purchasing power of a certain amount will be lower at a future date.
The concept of the time value of money is reflected or quantified with the help of discount rates. Discount rates help equalise the values of future cash flows to present terms, showing money’s earning potential over time. That’s why the discount rate is also referred to as the rate of return.
Thus, the discount rate is a reflection of the time value of money principle. It represents the rate at which future cash flows are discounted back to their present value, taking into account the earning potential of money over time.
Relation between Discount Rate and Internal Rate of Return
The discount rate, also known as the required rate of return or cost of capital, is the rate used to discount future cash flows back to their present value. It represents the opportunity cost of capital and reflects the investor’s required rate of return for undertaking the investment. The discount rate serves as a benchmark for determining whether an investment is financially viable.
The internal rate of return is the discount rate that considers an investment’s net present value (NPV) as zero. In other words, it is the rate at which the present value of cash inflows equals the present value of cash outflows. The IRR provides insight into the profitability of an investment project and is used to compare different investment opportunities.
The relationship between the discount rate and the internal rate of return is fundamental to investment analysis. When the discount rate is equal to the internal rate of return, the investment’s net present value is zero. This means that the project’s cash inflows exactly offset its cash outflows, resulting in no net gain or loss. In other words, the IRR represents the rate of return at which the investment breaks even.
If the discount rate is lower than the internal rate of return, the investment has a positive NPV and is considered financially attractive. Conversely, if the discount rate exceeds the internal rate of return, the investment has a negative NPV and may not be economically feasible.
Summing Up
The discount rate is a fundamental concept in finance that plays a crucial role in evaluating investment opportunities. It represents the minimum rate of return required by investors to compensate for the time value of money and the risk associated with an investment. From determining the weighted average cost of capital to assessing the net present value of projects, the discount rate guides decision-making processes in finance. Understanding its relationship with concepts like the risk-free rate, hurdle rate, and internal rate of return is essential for effective financial analysis and investment management. Ultimately, the discount rate serves as a cornerstone for evaluating the profitability and feasibility of investments in the dynamic world of finance.


